A solution based on the one from Richard is given below. I was not able to use Richard's original diagram but hope the one submitted is sufficient:

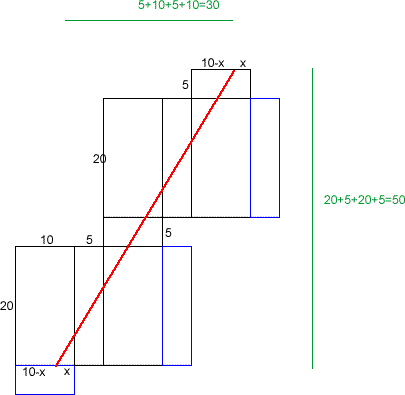
As the path of the ribbon around the box does not change direction when it reaches each edge, it can be drawn as a single straight line. As the ribbon crosses two faces of the box twice, a simple net of the box can show the path as a single straight line.

I therefore numbered the faces 1-6 and checked the order the ribbon went over them. This led to the diagram submitted, which I used to work out the length of the ribbon via Pythagoras' theorem.

The length of the ribbon is given by:
$$ \sqrt{(2 \times 5 + 2\times 10)^2 +(2 \times 5 + 2\times 20)^2}$$

By rearranging the above and substituting W, L and H for thelengths of the sides, the general expression for the length of the ribbon is therefore:

$$ 2\sqrt{(W+H)^2 + (L+H)^2}$$ or $$ 2\sqrt{W^2 + L^2 + 2H^2 + 2(WH + LH)} $$

A little more from Andrei - many thanks:

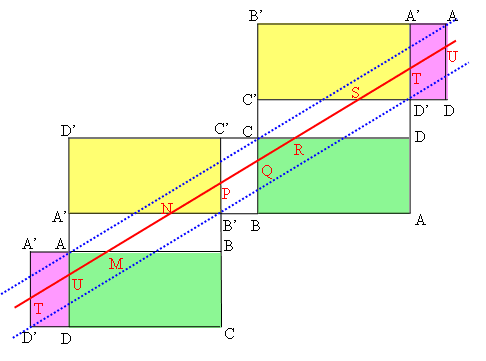
The condition of the problem is matched only when the red line passes through the same $U$, i.e. the dashed blue passes through the same $A$. So, the length of the ribbon is the distance $A$-$A$.

The blue dashed lines are the extreme positions of the ribbon, and between these positions the ribbon could glide. Each of these extreme positions has one of the two parallel lines reduced to a point.