Copyright © University of Cambridge. All rights reserved.
'Opening the Door' printed from https://nrich.maths.org/
Show menu
This resource is part of our Adventures with Complex Numbers collection
In the article Vanishing Roots, we posed the question:
"What if we could have the square root of a negative number?"
and introduced $i$ as a square root of $-1$, so $i^2=-1$.
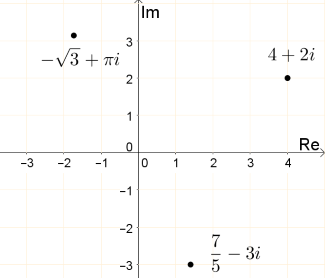
A complex number has a real part and an imaginary part, for example:
$4+ 2i$,
or $\frac{7}{5} - 3i$,
or $-\sqrt{3} + \pi i$
The complex number $a + bi$ has real part $a$ and imaginary part $b$.
In the same way that we use the number line to represent real numbers, we can use a coordinate plane to represent complex numbers. This plane is called the Argand diagram.
Adding and subtracting complex numbers is just the same as collecting like terms in algebra. For example,
$(4 + 2i) + \left(\frac{7}{5} - 3i \right) = \frac{27}{5} - i$
and
$(4 + 2i) - \left(\frac{7}{5} - 3i \right) = \frac{13}{5} + 5i$.
We have created the GeoGebra interactivity below for you to explore the questions that follow.
Use the Geogebra interactivity to find some pairs of complex numbers whose sum is a real number. What do you notice?
Can you explain it algebraically?
Use the Geogebra interactivity to find some pairs of complex numbers whose sum is an imaginary number. What do you notice?
Can you explain it algebraically?
In general, what would you need to add to $a + bi$ to get a real number? Or to get an imaginary number?
Now that you've been introduced to the world of complex numbers, you might like to start Strolling Along.
$4+ 2i$,
or $\frac{7}{5} - 3i$,
or $-\sqrt{3} + \pi i$
The complex number $a + bi$ has real part $a$ and imaginary part $b$.
In the same way that we use the number line to represent real numbers, we can use a coordinate plane to represent complex numbers. This plane is called the Argand diagram.
Adding and subtracting complex numbers is just the same as collecting like terms in algebra. For example,
$(4 + 2i) + \left(\frac{7}{5} - 3i \right) = \frac{27}{5} - i$
and
$(4 + 2i) - \left(\frac{7}{5} - 3i \right) = \frac{13}{5} + 5i$.
We have created the GeoGebra interactivity below for you to explore the questions that follow.
Use the Geogebra interactivity to find some pairs of complex numbers whose sum is a real number. What do you notice?
Can you explain it algebraically?
Use the Geogebra interactivity to find some pairs of complex numbers whose sum is an imaginary number. What do you notice?
Can you explain it algebraically?
In general, what would you need to add to $a + bi$ to get a real number? Or to get an imaginary number?
Now that you've been introduced to the world of complex numbers, you might like to start Strolling Along.